3D Lame Equation

 

Background

Let $\Omega \!\subset\!\mathbb{R}^3$ be a bounded, simply or multiply connected, domain in 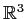with Lipschitz boundary $\Gamma$ occupying a homogeneous isotropic elastic solid that is characterized by the Lamé parameters $\lambda$ and $\mu.$ In linear elasticity, the Lamé equation which describes the static equilibrium of a deformable body in terms of the displacement vector $ \boldsymbol{u}\!\in\!\mathbb{R}^3$is defined as

$\displaystyle \mu\boldsymbol{\nabla}^2\boldsymbol{u}+(\lambda+\mu)\boldsymbol{\... ...symbol{\nabla}\!\boldsymbol{\cdot}\boldsymbol{u}+\boldsymbol{b}=\boldsymbol{0},$   

where $\boldsymbol{b}\!\in\!\mathbb{R}^3$ represents a body force prescribed on $\overline{\Omega }\!=\!\Omega \cup\Gamma .$ To deal with the Lamé equation, let where $\boldsymbol{n}$ be the unit normal to $\Gamma $ directed towards the exterior of $\Omega$ and let $ \boldsymbol{t}=\boldsymbol{n}\boldsymbol{\cdot}\boldsymbol{\sigma}$ be the traction vector on $\Gamma $ associated with the displacement $\boldsymbol{u},$ where the stress tensor$ \boldsymbol{\sigma}\in\mathbb{R}^{3\times3}$is introduced as

$\displaystyle \boldsymbol{\sigma}=\lambda \mathrm{div}\boldsymbol{u} \mathbf{... ...ldsymbol{\nabla}\boldsymbol{u}+ (\boldsymbol{\nabla}\boldsymbol{u})^{\tau}\big)$   

with $ \mathbf{I}_2$ denoting the symmetric second-order identity tensor on $ \mathbb{R}^3$ and the superscript "$ \tau$" representing the transpose symbol. A solution $ \boldsymbol{u}$ of the Lamé equation admits an integral representation, known as the Somigliana identity, expressed as

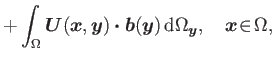
$\displaystyle \boldsymbol{u}(\boldsymbol{x})=\int_{\Gamma }\boldsymbol{U}(\bold... ...dsymbol{\cdot}\boldsymbol{u}(\boldsymbol{y}) {\rm d}\Gamma _{\!\boldsymbol{y}}$ $\displaystyle + \int_{\Omega }\boldsymbol{U}(\boldsymbol{x},\boldsymbol{y})\bol... ...dsymbol{y}) {\rm d}\Omega _{\boldsymbol{y}},\quad\boldsymbol{x}\!\in\!\Omega ,$   

where the kernels $ \boldsymbol{U}\!\in\!\mathbb{R}^{3\times3}$ and $ \boldsymbol{T}\!\in\!\mathbb{R}^{3\times3}$are given respectively as

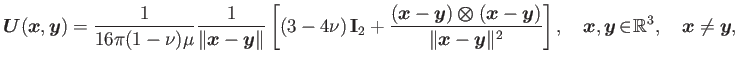
$\displaystyle \boldsymbol{U}(\boldsymbol{x},\boldsymbol{y})= \frac1{16\pi(1-\nu... ...bol{x},\boldsymbol{y}\!\in\!\mathbb{R}^3, \quad\boldsymbol{x}\ne\boldsymbol{y},$   

and

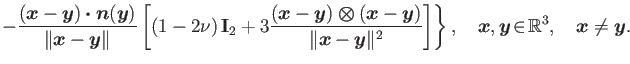
$\displaystyle \boldsymbol{T}(\boldsymbol{x},\boldsymbol{y})\!=$$\displaystyle \frac{-1}{8\pi(1-\nu)}\frac{1}{\Vert\boldsymbol{x}-\boldsymbol{y}... ...mbol{x}-\boldsymbol{y})}{\Vert\boldsymbol{x}-\boldsymbol{y}\Vert}\right]\right.$                                             $\displaystyle \left.\!-\frac{(\boldsymbol{x}-\boldsymbol{y})\boldsymbol{\cdot}\... ...mbol{x},\boldsymbol{y}\!\in\!\mathbb{R}^3,\quad\boldsymbol{x}\ne\boldsymbol{y}.$   

Here the elastic constant $ \nu=\lambda/(2(\lambda+\mu))$ is called the Poisson ratio of the solid $\Omega$ and$\Vert\boldsymbol{x}\Vert$ is the usual Euclidean norm in $\mathbb{R}^3$ defined as $\Vert\boldsymbol{x}\Vert=\sqrt{x_1^2+x_2^2+x_3^2}$. Moreover, it was established in [2] that the Newton potential admits a boundary representation as

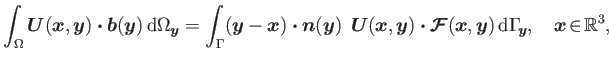
$\displaystyle \int_{\Omega }\boldsymbol{U}(\boldsymbol{x},\boldsymbol{y})\bolds... ...y}) {\rm d}\Gamma _{\!\boldsymbol{y}}, \quad\boldsymbol{x}\!\in\!\mathbb{R}^3,$   

where

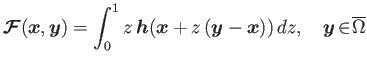
$\displaystyle \boldsymbol{\mathcal{F}}(\boldsymbol{x},\boldsymbol{y})=\int_{0}^... ...oldsymbol{y}-\boldsymbol{x})) dz,\quad \boldsymbol{y}\!\in\!\overline{\Omega }$   

with $ \boldsymbol{h}$ denoting an extension of the body force $ \boldsymbol{b}$ into any ball centered  at $ \boldsymbol{x}$ and containing $ \overline{\Omega }$ . In particular, a continuation $ \boldsymbol{h}$ of the body force $ \boldsymbol{b}$can be specified as

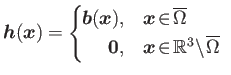
$\displaystyle \boldsymbol{h}(\boldsymbol{x}) = \left\{ \begin{array}{rl} \!\!\!... ...ymbol{x}\!\in\! \mathbb{R}^3\!\setminus\!\overline{\Omega } \end{array} \right.$   

This representation of the elastic Newton potential in term of surface integral allows a numerical solution of the Lamé equation that does not require a volume-fitted mesh.

 

Solution via a boundary element method

To approximately solve the Lamé equation via a Boundary Element Method (BEM), the surface $\Gamma$ is usually discretized into flat triangles $\Gamma_j$ using a mesh generation software (e.g. CUBIT).

\includegraphics[height=1.5in]{ct896g}

With reference to [1], the vector fields $\boldsymbol{u}$ and $ \boldsymbol{t}$ are assumed to have a polynomial variation over each triangle (boundary element)$\Gamma _j.$

Approaches:

  Collocation BEM

  Galerkin BEM

 

References

[1] S. Nintcheu Fata.
     Explicit expressions for three-dimensional boundary integrals in linear elasticity.
     J. Comput. Appl. Math., 235(15):4480-4495, 2011.
[2] S. Nintcheu Fata.
     Boundary integral approximation of volume potentials in three-dimensional linear elasticity.
     J. Comput. Appl. Math., 242(1):275-284, 2013.